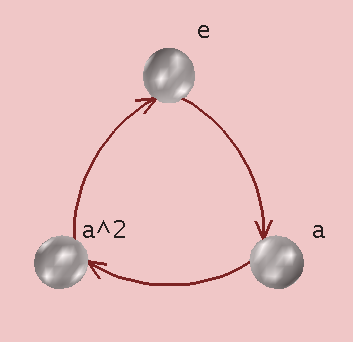
Cayley Diagrams

Cayley diagrams are a very potent but underused visualization technique, probably because creating them is difficult by hand. Group Explorer makes them easy to view and edit.

A Cayley diagram is a directed graph with a node for each element of the group, and a type of arrow for each group generator (Group Explorer distinguishes them via color). Cayley diagrams show the actions of the generators on all the group elements, and clearly communicate the group's structure and complexity (or lack thereof).

Cayley diagrams make it clear why cyclic groups are thus named.
This group is Z₃.

One can distinguish nonabelian groups easily by following arrows in different orders.
This is the smallest nonabelian group, S₃.

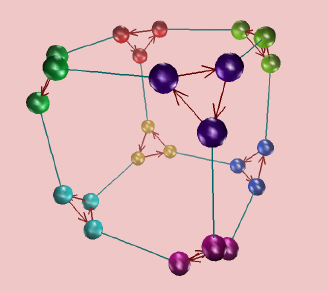Cayley diagrams of groups of symmetry can be laid out in a way that reveals the object they describe. The group of symmetries of the cube, S₄, is shown here. Note that because the cube can be rotated one-third of a circle about opposite corners, the individually colored cosets in the Cayley diagram show little copies of Z₃-like structure at each corner.

Both Cayley diagrams and multiplication tables can be used to show quotient group structure, but that goes beyond the scope of this introduction. (Search for "Organize by" in Group Explorer's help system.)

<< Prev: Multiplication tables

Next: Cycle graphs >>

You can download Group Explorer here, and the online help is far more in-depth than this introduction. Feel free to send comments and suggestions to the address below.

Contact Nathan Carter

Group Explorer 2.1

Introduction to Key Features

Cayley Diagrams